
The effect of one independent variable on average yield does not depend on the effect of the other independent variable (a.k.a. There is a difference in average yield by planting density. There is no difference in average yield at either planting density. There is a difference in average yield by fertilizer type. Two-way ANOVA hypothesesIn our crop yield experiment, we can test three hypotheses using two-way ANOVA: Null hypothesis (H 0) an additive two-way ANOVA) only tests the first two of these hypotheses. no interaction effect).Ī two-way ANOVA without interaction (a.k.a. The effect of one independent variable does not depend on the effect of the other independent variable (a.k.a.There is no difference in group means at any level of the second independent variable.There is no difference in group means at any level of the first independent variable.If the variance within groups is smaller than the variance between groups, the F-test will find a higher F-value, and therefore a higher likelihood that the difference observed is real and not due to chance.Ī two-way ANOVA with interaction tests three null hypotheses at the same time: The F-test is a groupwise comparison test, which means it compares the variance in each group mean to the overall variance in the dependent variable. How does the ANOVA test work?ĪNOVA tests for significance using the F-test for statistical significance. If one of your independent variables is categorical and one is quantitative, use an ANCOVA instead. You should have enough observations in your data set to be able to find the mean of the quantitative dependent variable at each combination of levels of the independent variables.īoth of your independent variables should be categorical. Planting densities 1 and 2 are levels within the categorical variable planting density. Fertilizer types 1, 2, and 3 are levels within the categorical variable fertilizer type. A level is an individual category within the categorical variable. It can be divided to find the average bushels per acre.Ī categorical variable represents types or categories of things. Bushels per acre is a quantitative variable because it represents the amount of crop produced. You can use a two-way ANOVA when you have collected data on a quantitative dependent variable at multiple levels of two categorical independent variables.Ī quantitative variable represents amounts or counts of things. Frequently asked questions about two-way ANOVA.
#Two way anova spss code how to#
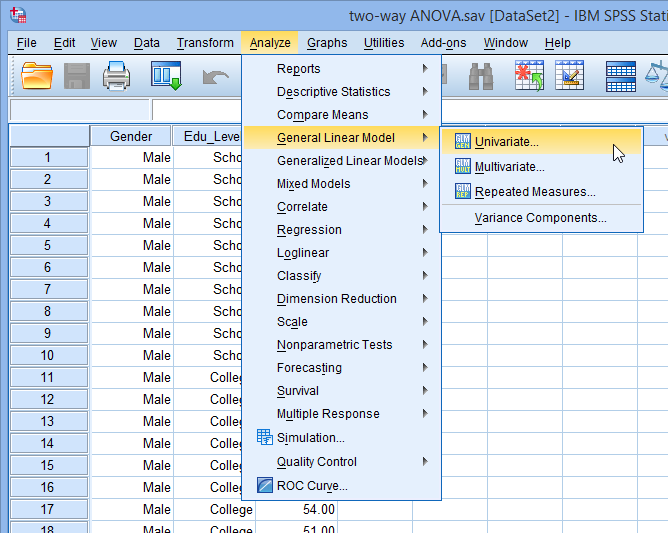
How to present the results of a a two-way ANOVA.Interpreting the results of a two-way ANOVA.The graphical results are shown in Output 39.3.5 through Output 39.3.7. Additionally, the PLOTS=MEANPLOT(CL) option specifies that confidence limits for the LS-means should also be displayed in the mean plot. The following statements reproduce the previous analysis with ODS Graphics enabled. If you enable ODS Graphics for the previous analysis, GLM also displays three additional plots by default:Īn interaction plot for the effects of disease and drugĪ plot of the adjusted pairwise differences and their significance levels Evidently, the main contribution to the significant drug effect is the difference between the 1/2 pair and the 3/4 pair. The multiple-comparison analysis shows that drugs 1 and 2 have very similar effects, and that drugs 3 and 4 are also insignificantly different from each other. Since the GLM procedure is interactive, you can accomplish this by submitting the following statements after the previous ones that performed the ANOVA. As the previous discussion indicates, Type III sums of squares correspond to differences between LS-means, so you can follow up the Type III tests with a multiple-comparison analysis of the drug LS-means.
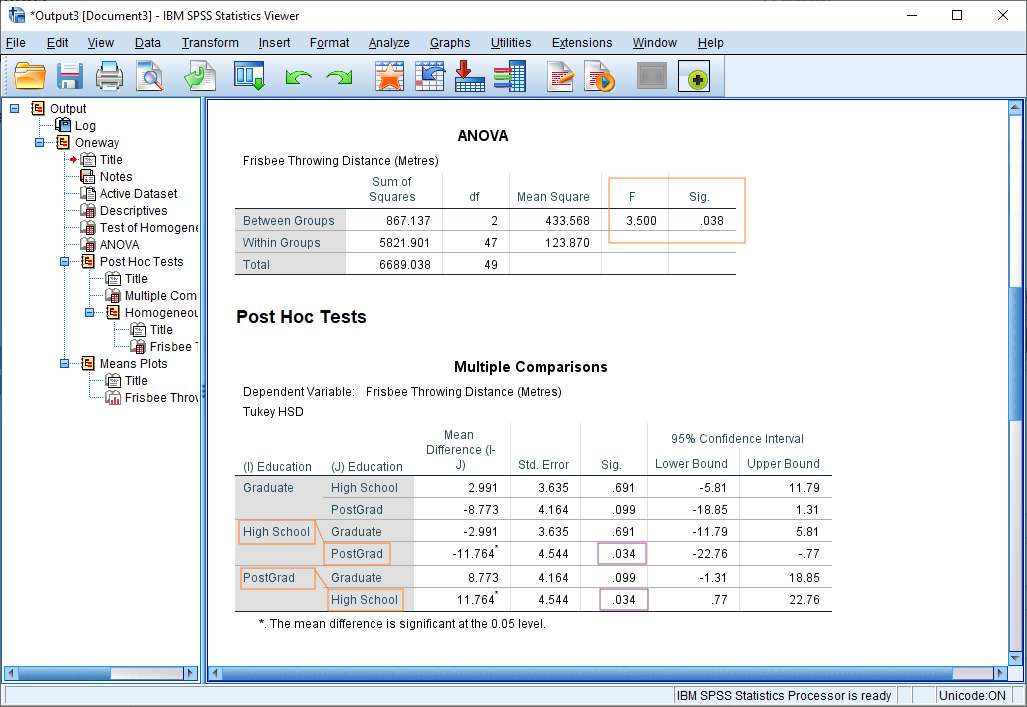
No matter which sum of squares you prefer to use, this analysis shows a significant difference among the four drugs, while the disease effect and the drug-by-disease interaction are not significant. Finally, the Type IV sum of squares is the same as the Type III sum of squares in this case, since there are data for every drug-by-disease combination. The Type III sum of squares measures the differences between predicted drug means over a balanced drug disease population-that is, between the LS-means for drug.
By contrast, the Type II sum of squares for drug measures the differences between arithmetic means for each drug after adjusting for disease. The Type I sum of squares for drug essentially tests for differences between the expected values of the arithmetic mean response for different drugs, unadjusted for the effect of disease. Note the differences among the four types of sums of squares.


 0 kommentar(er)
0 kommentar(er)
